Mathematics in the Primary School

Richard R. Skemp

• Publisher: Routledge
• Year: 2002
• ISBN:
  9781134974290
• Edition: 1
• Topic: Education
• Subtopic: Education General

National Curriculum guidelines emphasise knowledge, understanding and skills. The author, an internationally recognised authority, provides teachers with a clear explanation of these principles, and explains the relation between understanding and skills, and describes their application to the teaching of mathematics. The book contains numerous activities to show how mathematics can be learnt in the primary classroom with understanding and enjoyment, including:
* formation of mathematical concepts
* construction of knowledge
* contents and structure of primary mathematics

Part B

6

---

Making a start

How to use teaching as a learning experience for ourselves, as well as for our children

The model of intelligent learning which was offered in Part I is applicable to all ages. It may usefully be applied to our own learning of any subject for which the appropriate mix includes a substantial component of intelligent learning relative to habit learning. (Reminder: the building up of a collection of useful routines is not the same as habit learning, but a valuable component of intelligent learning.) This description undoubtedly fits the two learning tasks facing most readers—not to mention many mathematics teachers who are not readers!

The first task is ourselves to acquire, if we do not have it already, a well structured understanding of the foundation schemas of mathematics—those which children need to build during their early years of schooling. Surprising though this may seem, those who have a clear and reflective understanding of elementary mathematics are in a fortunate minority. Most people know what to do, but not why. And elementary does not necessarily mean simple, as I found when I turned my attention from mathematics at secondary school level and beyond to mathematics as taught in primary schools. The importance of conceptual analysis, leading to concept maps on which to base a well-structured collection of learning activities, has already been emphasized. It was this, for primary mathematics, which first opened my eyes to the conceptual complexity of many of the topics in primary mathematics which most of us use—and teach—intuitively.

The second task is to understand the processes of intelligent learning both at a theoretical level, and also in their practical application to the teaching of mathematics in our own classrooms, both day-to-day and long-term. To aim for anything less is, in my view, to be less than professional in one’s approach.

These goals will take more time to achieve than is available during most pre-service courses for teachers. But during this time it is possible to make a substantial beginning; and, more important, to find out how to continue learning ‘on the job’. And one does not have to travel far along this road to find that the journey can be both interesting and rewarding.

As a preliminary to applying the present model to these tasks, it will be useful to review the three modes of schema building and testing described in Chapter 4. These are reproduced below.

Figure 6.1

Experience has shown that a very good approach is a combination of modes 1 and 2, used in the ways described below. These may, of course, be adapted to the particular situation of the present reader. They are offered not as rules to be followed, but as a valuable combination of ways for building professional knowledge and skills.

At the end of this chapter are fourteen learning activities for children. They are offered here with an additional emphasis. Each of these activities embodies both a mathematical concept, and also one or more aspects of the theory. So by doing these with a group of children, both children and their teacher benefit. The children benefit by this approach to their learning of mathematics; and the teacher also has an opportunity to learn about the theory of intelligent learning by seeing it in action. Theoretical knowledge acquired in this way relates closely to classroom experience and to the needs of the classroom. It brings with it a bonus, since not only do the children benefit from this approach to mathematics, but it provides a good learning situation for teachers also. In this way we get ‘two for the price of one’, time-wise.

Observe and listen, reflect, discuss

In more detail, the method is as follows.

(i) As a preliminary to using them with children, you need to do the activities yourself, preferably with fellow-students or colleagues. At this stage it is useful to discuss their mathematical content. It is also good to go through the activity enough times to become fluent in the procedures, so that more of one’s attention can be free for observation of the children.

(ii) Do these activities with some children. Try not to be actively teaching every moment: allow the children time to think for themselves. This will also give you time to observe and listen.

(iii) Reflect on your observations, and make notes.

(iv) Discuss your experiences and observations with a colleague, fellow-student, or tutor, according to circumstances. In these discussions it is useful to try to relate the observations, and your inferences from them, to the parent model. This provides a language for talking about the learning processes involved, and helps to relate the particular instances being discussed to an integrated and more general knowledge structure.

When some of these activities were first used in the way described here, the results were very encouraging. Experienced teachers on an in-service course said to the organizing tutor (not the present writer) that they had learnt more from these observations than from all the books they had read at college. In Canada, a teacher said ‘It’s as though the children’s thinking was out there on the table’.

This was not only encouraging, but beyond what had been expected. Subsequent reflection and analysis in terms of the present model, and particularly in terms of the ideas discussed in Chapter 5, have suggested the following explanation.

What makes mathematics so powerful a tool for understanding, predicting, and sometimes controlling events in the physical world is the fact that it provides such an accurate and multi-purpose model of the physical world. But this correspondence is the one shown in Figure 6.2 by the lower arrow, not the upper one: between the deep structures of mathematics and the physical world, not the surface structures and the physical world. So when we give children materials to manipulate which embody these deep structures, we are doing two very important things. We are letting them experience, in simple examples, the power of mathematics to organize and understand the physical world in their own here-and-now. And when children are working with these manipulatives, they reveal the deep structures of their thinking more clearly than they do by words alone, still less by written symbols. Furthermore, the dominance of the deep structures in these activities helps to ensure that their spoken symbolism expresses these, rather than verbally memorized rules at a surface level. Children can sometimes be very articulate about mathematics in learning situations of this kind.

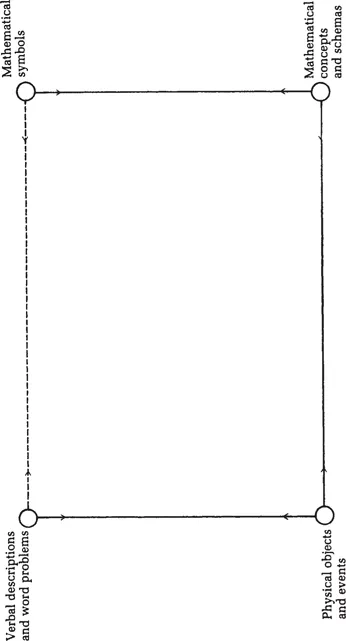
Figure 6.2

Some observations which have revealed children’s thinking

Here are two examples. Readers who use the activities at the end of this chapter in the ways suggested will be able to provide other examples for themselves.

1 A colleague¹ was working on addition with three young children in a reception class. She asked them if they could say what five plus four made. All gave the same answer, nine: but each used a different method.

The first counted out 5 counters, then counted 4 more, and then counted all of them beginning from 1. From this we may deduce that he knew what the question meant, and knew how to use mode 1 schema building to find out something which he didn’t yet have as a permanent part of his mental model. So he had a way of learning which did not depend on being told the answer, and which related his mathematics to the physical world.

The second used her fingers, and was seen to be counting on from 5, 4 more. This child still needed concrete support for her thinking, but was at a more advanced stage in addition. Counting on implies at least an intuitive awareness that the first set is included in the larger set formed by the union of the two sets: so it does not need to be counted again. Put like this, we can see that the step from counting all to counting on is more sophisticated than perhaps we give children credit for.

The third child just looked at the ceiling. My colleague said ‘I could see how the other two got their answers, but I couldn’t see how you did. Can you tell me?’ He replied ‘Five add five is ten. Four is one less than five, so the answer is one less: nine.’ In my view, this is both good thinking and good explaining. This child was working at an abstract level, involving an inference between two relationships. He was also able to reflect on his own thinking, and was highly articulate about this. Though his chronological age was about the same as that of the other two, mathematically he was far ahead.

2 I was visiting a school in company with an advisory teacher. We were in different classrooms, but met for the lunch break. She said to me ‘I’m thrilled with Capture. I’ve just seen two children go from concrete to abstract thinking.’²

This game is described in detail at the end of this chapter. Briefly, it is a game for two children, using two number tracks side by side. Each rolls a die, and puts this number of cubes on her own number track. The one with the larger number captures the difference. After a while, these children had stopped using the number tracks. If (say) one threw 5 and the other 3, the first one just took 2 of the other’s cubes.

Both these observations support the view that the use of ...

Table of contents

• Cover Page
• Half Title page
• Series page
• Title Page
• Copyright Page
• Contents
• Acknowledgements
• Prologue: Relational understanding and instrumental understanding
• Part A
• Part B
• Index